does anybody know how to write for example this formala into the expr object: c=(((c²+c)²+c)²+c)²+c ? its the fourth iteration of a fractal. this is the first iteration: c=c and this is the second c=c²+c. my second question is if there is a formula to get the first, secont, third iteration etc.. i hope my mathematical terms are correct and understandable

@Jona I tried to implement the manit patch into your sequencer too but couldn't get it to show anything! Would you mind sharing the patch?

Perhaps there are other, more varying formulas that lend themselves better to musical explorations in this fashion. In Elaine's paper it is discussed how to use complex chaotic functions, so perhaps the manit patch could be adapted for other formulas?

@weightless here is the patch: manit-seq.zip

@Jona Thanks a lot! I will have a look and maybe learn something from how you plot the values with the data structure.

@weightless this is the part of the patch that plots the values. it chooses the $0-draw element of the $0-array array and then sets the x and y value. each step bangs every existing element. c is the color of the element. 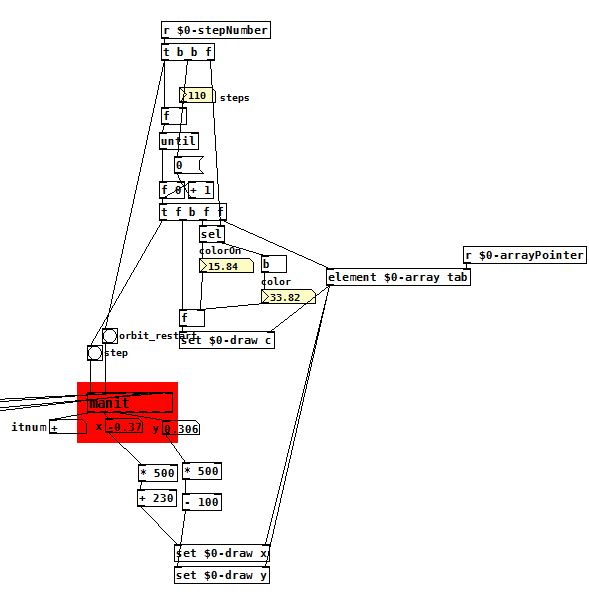if you have a question about the plotting i will try to answer.

and i'm not exactly sure if the 'classic mandelbrot-set series' yields any musically interesting results, but
i am quite sure that it only produces usable results for carefully chosen values of c (on the border of the mandelbrot set where it neither converges nor diverges, at least not so quick...).

maybe simple real-valued recursive functions are more fun. the idea is to blow up a value (multiplying, adding constants, whatever), then wrap it to a range, and feed it to the next iteration. like the classic gingerbreadman, hopalong etc. fractals. linke in the attached example. fractalmelody.pd

@mnb thanks, nice patch. that sounds very interesting. i will have to learn about that.

@mnb the recursion trick with the sine function is nice. i tried to create a longer slow development with your formula (its quite repetetive in the beginning):

@Jona The dream-seq is now also working:

dream-seq.pd (updated)

The formula is the "Kings Dream" fractal taken from http://softology.com.au/tutorials/attractors2d/tutorial.htm. There are some more interesting formulas on that page.

Some parameters give decent results. For some parameter combinations it is better to turn motion off so it would not converge against a less interesting figure.

Update:
Now there is a toggle "symmetry". When it is checked the parameters on the left will also set the parameters on the right. The right ones are still free. Also changed the plot to bezier curve. It doesn't plot through the nodes, but is still nice.

@ingox very nice. more possibilities than i thought